You had me at 'magnets'

There is never enough magnets to play with (and learn some science along the way).

Admit it – you love magnets! Their ability to push and attract each other from distance feels like magic. Yes, science tells you what this is, but still … it's like “the force” from Star Wars. No surprise, then, that kids love to play with magnets. And this enjoyment is what we can exploit to introduce concepts from mathematics, physics, and chemistry into seemingly simple games.

Note that examples I show are not for very small kids who could swallow the magnets, which can be extremely dangerous. This is for early elementary schools kids or older. Examples also depend on the previous concepts that you have shown to the kids.

Today I will talk about geometry of magnet configurations, which is applicable in physics and chemistry. You might also want to check other examples:

• if you tried to make crystals at home, then a handful of small ball magnets can be used for showing the basic atomic idea behind crystal growth,

• if you talked about DNA (or even extract it from plants), then you can demonstrate using magnets how DNA can extend into a very long chain while being packed into a tiny space of cell nucleus,

• if you played with home chemistry, then you can use magnets to show analogs of temperature change in chemical reactions,

• or if you talked about electric currents and semiconductors, magnets can be used for explaining the concept of “holes” as particles.

When dealing with magnets, it is useful to see the magnet strength at different locations around the magnet. Such a map within the volume surrounding a magnet is called the magnetic field. You can actually see this field, i.e. a 3D map, with a little trick using a bottle of baby oil and specks of steel wool (follow THIS recipe).

In general, the old fashioned method of iron dust on a paper will help you reveal the shape of magnetic field. I use a home-made version of this where the iron specks are trapped between two Plexiglas plates (if iron dust ends up on your magnet, it is really hard to remove it). Anther great option is to purchase magnetic field viewing film – a foil that changes its colors when exposed to magnetic fields. Here is what you get if you trace the field around a small ball magnet:

Magnets have two poles – north and south. There is no magnet with only a single pole. The reason for that remains one of the Universe's mysteries. The red arrow shows the direction connecting them. In this exercise it is not important which is which, we only care to keep the arrow oriented always in the same way.

The shape of magnetic field visible in this case is typical of magnets. We call it a “dipole field”, since it is a result of two poles interacting with each other. A dipole field is a concept that you encounter daily on a big scale: the whole Earth is surrounded by a big magnetic dipole. Also, radio antennas often emit in this configuration, including the antenna in your cell phone. Chemists see it in molecules where the electric charge is unevenly distributed within molecules (e.g., water molecule), which boosts interaction between molecules by electric force.

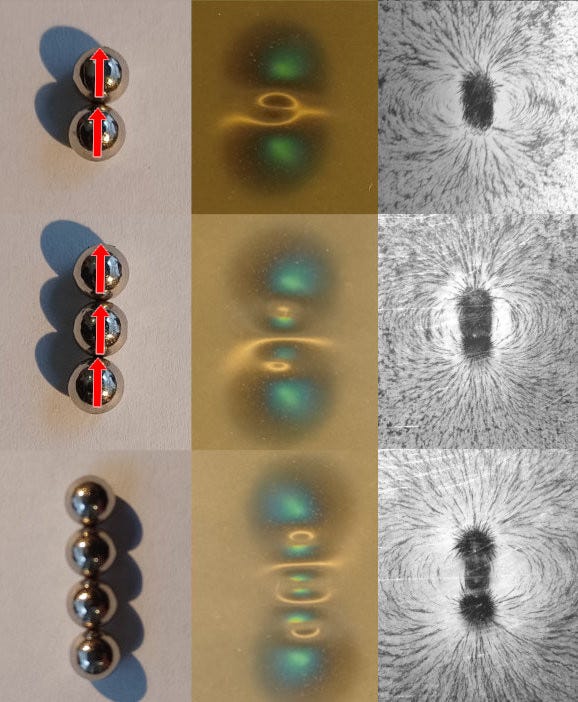
Now we start wondering what happens if we build a magnetic chain. First we put two magnets together. Then we add another one, and another one, …

… and finally we create a very long chain. How does that affect the shape of magnetic field?

Here we reached an interesting point. Notice how the magnetic field along the chain is constrained to the space very close to the magnets, like a magnetic tube, but at the chain ends the field goes almost radially far away. Magnetic field is enhanced on these ends, where the field lines must find the other pole over a large distance.

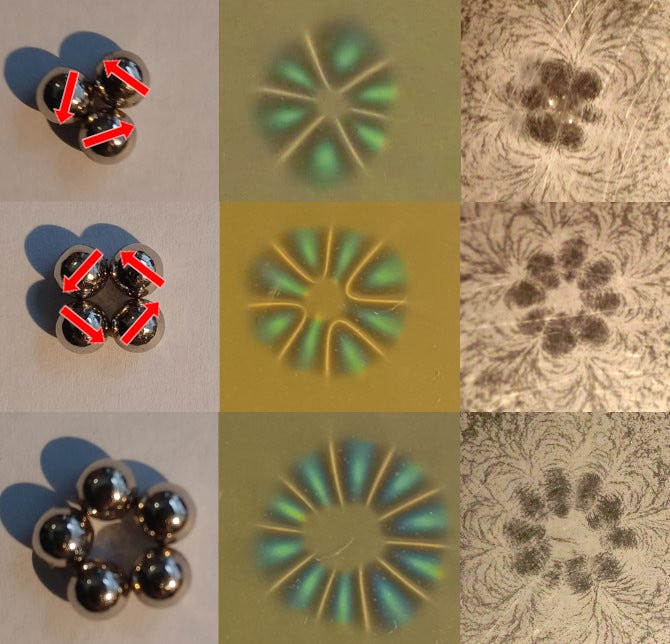
What about closing the chain into a loop? A long loop creates a weak magnet all over the loop because there is no end to it any more. But a more interesting configuration happens when we use a small number of magnets in a loop. You will start to see so called multipole magnets – configurations exploited in some type of electromotors, or in particle accelerators.

Their main feature is a beautiful symmetry of the magnetic field loops formed around them. The magnets spontaneously form these configurations when you force them to come close to each other.

When you put six ball magnets in a loop, an interesting question opens up. Six balls form the central space big enough to add another ball at the loop center. This additional central magnet will break up the perfect symmetry. How will this affect the magnetic field configuration? Here is the answer:

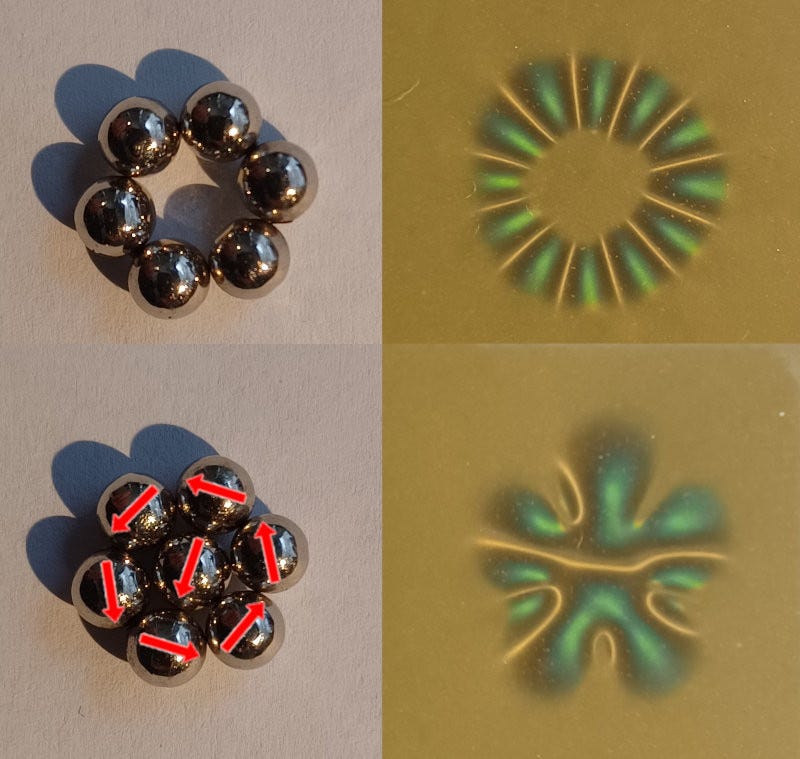
The result is quite complicated and difficult to predict intuitively. The nice symmetric configuration is completely gone because of this one additional magnet. Let's try this with a double and triple loop of magnets:

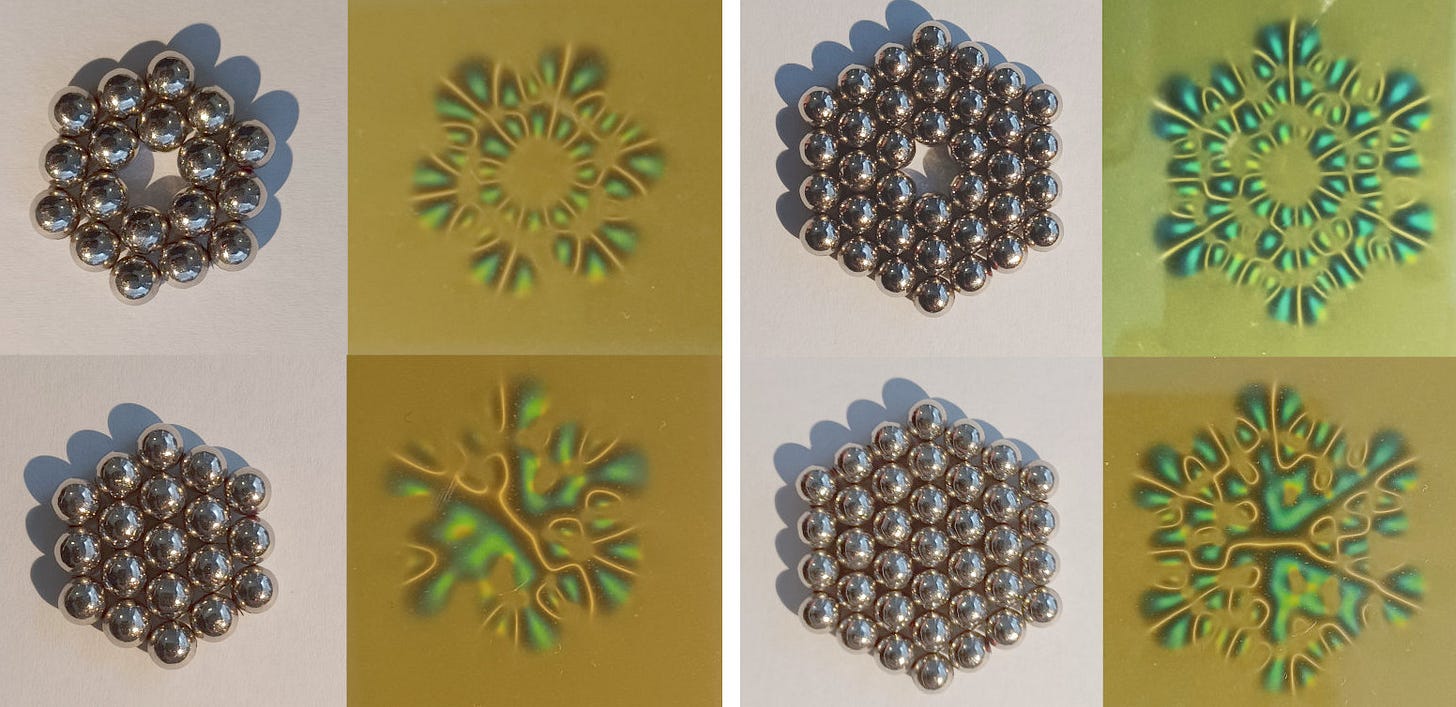
Again, one extra magnet put in the center and the symmetry is broken. What if we make a much bigger sheet of magnets oriented in concentric loops?

The sheet is now big enough to surpass the size of central perturbation.

Does this mean that an arbitrarily big layer of magnets, i.e. dipoles, can exist in a stable configuration only as such concentric rings, where the center is always perturbed? Many materials have dipoles on the atomic or molecular level, so this question is quite important.

Interestingly, if you play with ball magnets a little bit more, you will discover that magnets can form a stable infinite sheet in two other ways. Take long chains and bring them together side by side.

In one configuration all the magnets point to the same direction. In the other configuration they alternate between two directions. You can see how the position of balls differs between these two options. The mechanism pushing magnets into these stable configurations is the force between poles. Magnets try to minimize the distance between the opposite poles to balance the repulsion between the same poles. The final result of this minimization is revealed by the viewing film, which shows the same regular pattern of north-south pairs in both configurations, even though the relative position of balls is different.

Now we move our focus on one important detail: sheet edges. Iron dust detects a large difference between the two configurations. The sheet with alternating magnet directions has the ends of chains forming an edge with alternating poles. This helps with localizing the magnetic field, since the field needs to stretch only to the first neighbor to close itself between the north-south pairs.

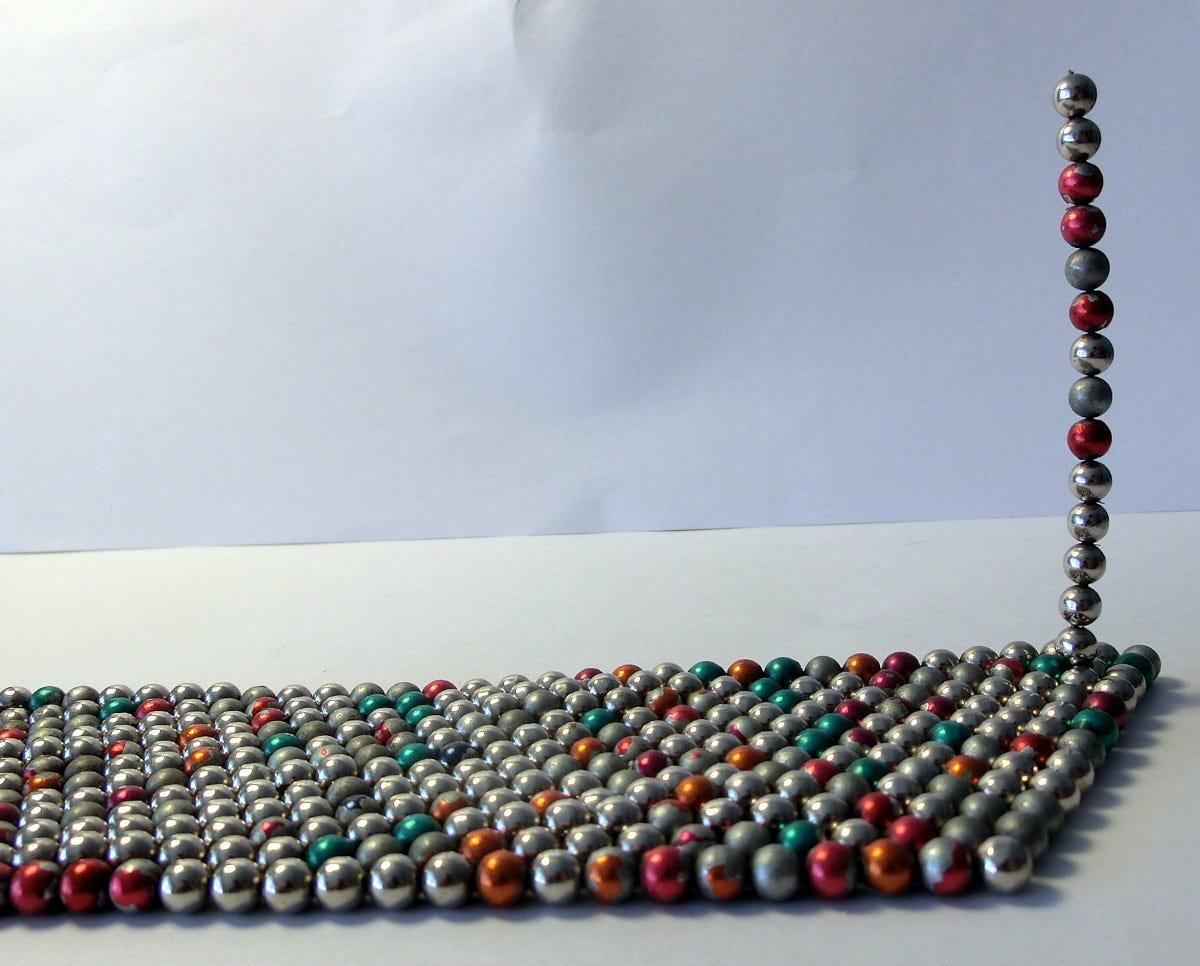
An entirely different edge at the end of chains is formed when all magnets point toward the same direction. The edge is packed with magnets oriented in the same direction, forming a strong magnetic force as a collective. The strong field lines extend far away as they try to close the north-south configuration.

You can play fun games with adding magnets on the top of such edge. Magnets will easily form a vertical chain defying gravity.

Understanding the weirdness of edges is important because the surface of materials (including liquids) on the atomic level is also a weird world. Atoms on the surface are exposed only partially to the electric forces that keep them attached to the material. This is why special branches of science, called surface physics and surface chemistry, exist to study surfaces (interfaces between materials, including solid-liquid, solid-gas, solid-vacuum, liquid-gas, etc.).

Another interesting game is joining sheets vertically together into a 3D stack. If we create an additional magnetic sheet with the opposite polarity to the previous one, then we can join them and create a very stable two-layer configuration. Notice how the strong magnetic field on the edge is now tamed because the opposite poles sit on the top of each other.

Obviously, one single layer of magnets displays different properties than a multi-layer structure. The same happens in nature, were the story of graphene first comes to my mind. Graphene is a single, one atomic layer of graphite. One would think that such an isolated layer would easily break. After all, it is just a single layer of atoms. But in 2004 physicist discovered a simple way how to isolate graphene and it turned out that it has some remarkable properties and stability.

The two layer magnetic structure is also fun to explore by bending it. If you slightly deform it, you will feel how it “clicks” into a stable twisted configuration. Notice what happens – magnets found new pairs of opposite polarity and left single magnets at the kinks unpaired. Real materials can show a similar behavior on the atomic level.

Magnets can also be put into a ring structure, where we pack loops on the top of each other. The rotational shift between stacked loops is again driven by the magnet orientation.

Or we can make a magnetic tube by rolling a magnetic chain into a 3D spiral. You will notice that the shape of the tube walls depends on whether you use a single chain or a double chain. The magnets lock into positions in different ways, which leads to different geometries of the tube walls.

We can find a similar diversity in material science, for example with carbon nanotubes. Imagine the previously mentioned graphene folded onto itself to form a tube. The physical and chemical properties of such tubes depend on their geometry.